QUIZ
Q1) For a spherical concave mirror, virtual images are formed when the object is located
A. between F and C
B. beyond C
C. at C
D. inside F
Answer) D.
Q2) Which of the following is not true when an image is formed by an object located between C and F of a concave mirror?
A. Negative magnification
B. Negative image distance
C. Inverted image
D. Enlarged image
Answer) C.
If you look at the surface of a shiny spoon, you will notice that your reflection is different from what you see in a plane mirror. The spoon acts as a curved mirror, with one side curved inward and the other curved outward. The properties of curved mirrors and the images that they form depend on the shape of the mirror and the object’s position.
Concave Mirrors
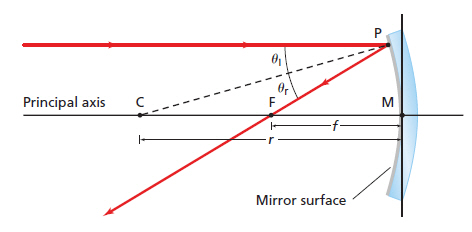
The inside surface of a shiny spoon, the side that holds food, acts as a concave mirror. A concave mirror has a reflective surface, the edges of which curve toward the observer. Properties of a concave mirror depend on how much it is curved. Figure 1 shows how a spherical concave mirror works. In a spherical concave mirror, the mirror is shaped as if it were a section of a hollow sphere with an inner reflective surface. The mirror has the same geometric center, C, and radius of curvature, r, as a sphere of radius, r. The line that includes line segment CM is the principal axis, which is the straight line perpendicular to the surface of the mirror that divides the mirror in half. Point M is the center of the mirror where the principal axis intersects the mirror.
When you point the principal axis of a concave mirror toward the Sun, all the rays are reflected through a single point. You can locate this point by moving a sheet of paper toward and away from the mirror until the smallest and sharpest spot of sunlight is focused on the paper. This spot is called the focal point of the mirror, the point where incident light rays that are parallel to the principal axis converge after reflecting from the mirror.
The Sun is a source of parallel light rays because it is very far away. All of the light that comes directly from the Sun must follow almost parallel paths to Earth, just as all of the arrows shot by an archer must follow almost parallel paths to hit within the circle of a bull’s-eye.
When a ray strikes a mirror, it is reflected according to the law of reflection. Figure 1 shows that a ray parallel to the principal axis is reflected and crosses the principal axis at point F, the focal point. F is at the halfway point between M and C. The focal length, f, is the position of the focal point with respect to the mirror along the principal axis and can
be expressed as f r/2. The focal length is positive for a concave mirror.
Figure 2. The real image, as seen by the unaided eye (a). The unaided eye cannot see the real image if it is not in a location to catch the rays that form the image (b). The real image as seen on a white opaque screen (c).
Graphical Method of Finding the Image
You have already drawn rays to follow the path of light that reflects off plane mirrors. This method is even more useful when applied to curved mirrors.
Not only can the location of the image vary, but so can the orientation and size of the image. You can use a ray diagram to determine properties of an image formed by a curved mirror. Figure 2 shows the formation of a real image, an image that is formed by the converging of light rays. The image is inverted and larger than the object. The rays actually converge at the point where the image is located. The point of intersection, I, of the two reflected rays determines the position of the image. You can see the image floating in space if you place your eye so that the rays that form the image fall on your eye, as in Figure 2a. As Figure 2b shows, however, your eye must be oriented so as to see the rays coming from the image location. You cannot look at the image from behind. If you were to place a movie screen at this point, the image would appear on the screen, as shown in Figure 2c. You cannot do this with virtual images.
To more easily understand how ray tracing works with curved mirrors, you can use simple, one-dimensional objects, such as the arrow shown in Figure 3a. A spherical concave mirror produces an inverted real image if the object position, do, is greater than twice the focal length, f. The object is then beyond the center of curvature, C. If the object is placed between the center of curvature and the focal point, F, as shown in Figure 3b, the image is again real and inverted. However, the size of the image is now greater than the size of the object.
Figure 3. When the object is farther from the mirror than C, the image is a real image that is inverted and smaller compared to the object (a). When the object is located between C and F, the real image is inverted, larger than the object, and located beyond C (b).
Magnification Another property of a spherical mirror is magnification, m, which is how much larger or smaller the image is relative to the object.
In practice, this is a simple ratio of the image height to the object height. Using similar-triangle geometry, this ratio can be written in terms of image position and object position.
Image position is positive for a real image when using the preceding equations. Thus, the magnification is negative, which means that the image is inverted compared to the object. If the object is beyond point C, the absolute value of the magnification for the real image is less than 1.
This means that the image is smaller than the object. If the object is placed between point C and point F, the absolute value of the magnification for the real image is greater than 1. Thus, the image is larger than the object.
Figure 4. When an object is located between the focal point and a spherical concave mirror, a virtual image that is upright and larger compared to the object is formed behind the mirror (a), as shown with the stack of blocks (b). What else do you see in this picture?
Virtual Images with Concave Mirrors
You have seen that as an object approaches the focal point, F, of a concave mirror, the image moves farther away from the mirror. If the object is at the focal point, all reflected rays are parallel. They never meet, therefore, and the image is said to be at infinity, so the object could never be seen. What happens if the object is moved even closer to the mirror? What do you see when you move your face close to a concave mirror?
The image of your face is right-side up and behind the mirror. A concave mirror produces a virtual image if the object is located between the mirror and the focal point, as shown in the ray diagram in Figure 4a. Again, two rays are drawn to locate the image of a point on an object. As before, ray 1 is drawn parallel to the principal axis and reflected through the focal point. Ray 2 is drawn as a line from the point on the object to the mirror, along a line defined by the focal point and the point on the object.
At the mirror, ray 2 is reflected parallel to the principal axis. Note that ray 1 and ray 2 diverge as they leave the mirror, so there cannot be a real image. However, sight lines extended behind the mirror converge, showing that the virtual image forms behind the mirror.
When you use the mirror equation to solve problems involving concave mirrors for which an object is between the mirror and the focal point, you will find that the image position is negative. The magnification equation gives a positive magnification greater than 1, which means that the image is upright and larger compared to the object, like the image in Figure 4b.
Figure 5. A convex mirror
always produces virtual images
that are upright and smaller
compared to the object.
Convex Mirrors
In the first part of this chapter, you learned that the inner surface of a shiny spoon acts as a concave mirror. If you turn the spoon around, the outer surface acts as a convex mirror, a reflective surface with edges that curve away from the observer. What do you see when you look at the back of a spoon? You see an upright, but smaller image of yourself.
Properties of a spherical convex mirror are shown in Figure 5. Rays reflected from a convex mirror always diverge. Thus, convex mirrors form virtual images. Points F and C are behind the mirror. In the mirror equation, f and di are negative numbers because they are both behind the mirror.
The ray diagram in Figure 5 represents how an image is formed by a spherical convex mirror. The figure uses two rays, but remember that there are an infinite number of rays. Ray 1 approaches the mirror parallel to the principal axis. The reflected ray is drawn along a sight line from F through the point where ray 1 strikes the mirror. Ray 2 approaches the mirror on a path that, if extended behind the mirror, would pass through F.
The reflected part of ray 2 and its sight line are parallel to the principal axis. The two reflected rays diverge, and the sight lines intersect behind the mirror at the location of the image. An image produced by a single convex mirror is a virtual image that is upright and smaller compared to the object.
The magnification equation is useful for determining the apparent dimensions of an object as seen in a spherical convex mirror. If you know the diameter of an object, you can multiply by the magnification fraction to see how the diameter changes. You will find that the diameter is smaller,
as are all other dimensions. This is why the objects appear to be farther away than they actually are for convex mirrors.
Figure 6. Convex mirrors produce images that are smaller than the objects. This increases the field of view for observers.
Field of view
It may seem that convex mirrors would have little use because the images that they form are smaller than the objects. However, this property of convex mirrors does have practical uses. By forming smaller images, convex mirrors enlarge the area, or field of view, that an observer sees, as shown in Figure 6. Also, the center of this field of view is visible from any angle of an observer off the principal axis of the mirror;
thus, the field of view is visible from a wide perspective. For this reason, convex mirrors often are used in cars as passenger-side rearview mirrors.
Mirror Comparison
How do the various types of mirrors compare? Table 1 compares the properties of single-mirror systems with objects that are located on the principal axis of the mirror. Virtual images are always behind the mirror, which means that the image position is negative. When the absolute value of a magnification is between zero and one, the image is smaller than the object. A negative magnification means the image is inverted relative to the object. Notice that the single plane mirror and convex mirror produce only virtual images, whereas the concave mirror can produce real images or virtual images. Plane mirrors give simple reflections, and convex mirrors expand the field of view. A concave mirror acts as a magnifier when an object is within the focal length of the mirror.
A. between F and C
B. beyond C
C. at C
D. inside F
Answer) D.
Q2) Which of the following is not true when an image is formed by an object located between C and F of a concave mirror?
A. Negative magnification
B. Negative image distance
C. Inverted image
D. Enlarged image
Concave and Convex Mirrors Simulation
When the next simulation is not visible, please refer to the following link.
If you look at the surface of a shiny spoon, you will notice that your reflection is different from what you see in a plane mirror. The spoon acts as a curved mirror, with one side curved inward and the other curved outward. The properties of curved mirrors and the images that they form depend on the shape of the mirror and the object’s position.
Concave Mirrors
The inside surface of a shiny spoon, the side that holds food, acts as a concave mirror. A concave mirror has a reflective surface, the edges of which curve toward the observer. Properties of a concave mirror depend on how much it is curved. Figure 1 shows how a spherical concave mirror works. In a spherical concave mirror, the mirror is shaped as if it were a section of a hollow sphere with an inner reflective surface. The mirror has the same geometric center, C, and radius of curvature, r, as a sphere of radius, r. The line that includes line segment CM is the principal axis, which is the straight line perpendicular to the surface of the mirror that divides the mirror in half. Point M is the center of the mirror where the principal axis intersects the mirror.
Figure 1. The focal point r of a spherical concave mirror is located halfway between the center of curvature and the mirror surface. Rays entering parallel to the principal axis are reflected to converge at the focal point, F.
When you point the principal axis of a concave mirror toward the Sun, all the rays are reflected through a single point. You can locate this point by moving a sheet of paper toward and away from the mirror until the smallest and sharpest spot of sunlight is focused on the paper. This spot is called the focal point of the mirror, the point where incident light rays that are parallel to the principal axis converge after reflecting from the mirror.
The Sun is a source of parallel light rays because it is very far away. All of the light that comes directly from the Sun must follow almost parallel paths to Earth, just as all of the arrows shot by an archer must follow almost parallel paths to hit within the circle of a bull’s-eye.
When a ray strikes a mirror, it is reflected according to the law of reflection. Figure 1 shows that a ray parallel to the principal axis is reflected and crosses the principal axis at point F, the focal point. F is at the halfway point between M and C. The focal length, f, is the position of the focal point with respect to the mirror along the principal axis and can
be expressed as f r/2. The focal length is positive for a concave mirror.
Figure 2. The real image, as seen by the unaided eye (a). The unaided eye cannot see the real image if it is not in a location to catch the rays that form the image (b). The real image as seen on a white opaque screen (c).
You have already drawn rays to follow the path of light that reflects off plane mirrors. This method is even more useful when applied to curved mirrors.
Not only can the location of the image vary, but so can the orientation and size of the image. You can use a ray diagram to determine properties of an image formed by a curved mirror. Figure 2 shows the formation of a real image, an image that is formed by the converging of light rays. The image is inverted and larger than the object. The rays actually converge at the point where the image is located. The point of intersection, I, of the two reflected rays determines the position of the image. You can see the image floating in space if you place your eye so that the rays that form the image fall on your eye, as in Figure 2a. As Figure 2b shows, however, your eye must be oriented so as to see the rays coming from the image location. You cannot look at the image from behind. If you were to place a movie screen at this point, the image would appear on the screen, as shown in Figure 2c. You cannot do this with virtual images.
To more easily understand how ray tracing works with curved mirrors, you can use simple, one-dimensional objects, such as the arrow shown in Figure 3a. A spherical concave mirror produces an inverted real image if the object position, do, is greater than twice the focal length, f. The object is then beyond the center of curvature, C. If the object is placed between the center of curvature and the focal point, F, as shown in Figure 3b, the image is again real and inverted. However, the size of the image is now greater than the size of the object.
Mathematical Method of Locating the Image
The spherical mirror model can be used to develop a simple equation for spherical mirrors. You must use the paraxial ray approximation, which states that only rays that are close to and almost parallel with the principal axis are used to form an image. Using this, in combination with the law of reflection, leads to the mirror equation, relating the focal length, f, object position, do, and image position, di, of a spherical mirror.
Mirror Equation
$\ \frac{1}{f}=\frac{1}{d_i}+\frac{1}{d_0}$
The reciprocal of the focal length of a spherical mirror is equal to the sum of the reciprocals of the image position and the object position.
|
When using this equation to solve problems, it is important to remember that it is only approximately correct. It does not predict spherical aberration, because it uses the paraxial ray approximation. In reality, light coming from an object toward a mirror is diverging, so not all of the light is close to or parallel to the axis. When the mirror diameter is small relative to the radius of curvature to minimize spherical aberration, this
equation predicts image properties more precisely.
In practice, this is a simple ratio of the image height to the object height. Using similar-triangle geometry, this ratio can be written in terms of image position and object position.
Magnification m
$\ m=\frac{h_i}{h_0}=\frac{-d_i}{d_0}$ The magnification of an object by a spherical mirror, defined as the image height divided by the object height, is equal to the negative of the image position, divided by the object position. |
Image position is positive for a real image when using the preceding equations. Thus, the magnification is negative, which means that the image is inverted compared to the object. If the object is beyond point C, the absolute value of the magnification for the real image is less than 1.
This means that the image is smaller than the object. If the object is placed between point C and point F, the absolute value of the magnification for the real image is greater than 1. Thus, the image is larger than the object.
Figure 4. When an object is located between the focal point and a spherical concave mirror, a virtual image that is upright and larger compared to the object is formed behind the mirror (a), as shown with the stack of blocks (b). What else do you see in this picture?
Virtual Images with Concave Mirrors
You have seen that as an object approaches the focal point, F, of a concave mirror, the image moves farther away from the mirror. If the object is at the focal point, all reflected rays are parallel. They never meet, therefore, and the image is said to be at infinity, so the object could never be seen. What happens if the object is moved even closer to the mirror? What do you see when you move your face close to a concave mirror?
The image of your face is right-side up and behind the mirror. A concave mirror produces a virtual image if the object is located between the mirror and the focal point, as shown in the ray diagram in Figure 4a. Again, two rays are drawn to locate the image of a point on an object. As before, ray 1 is drawn parallel to the principal axis and reflected through the focal point. Ray 2 is drawn as a line from the point on the object to the mirror, along a line defined by the focal point and the point on the object.
At the mirror, ray 2 is reflected parallel to the principal axis. Note that ray 1 and ray 2 diverge as they leave the mirror, so there cannot be a real image. However, sight lines extended behind the mirror converge, showing that the virtual image forms behind the mirror.
When you use the mirror equation to solve problems involving concave mirrors for which an object is between the mirror and the focal point, you will find that the image position is negative. The magnification equation gives a positive magnification greater than 1, which means that the image is upright and larger compared to the object, like the image in Figure 4b.
Convex Mirrors
In the first part of this chapter, you learned that the inner surface of a shiny spoon acts as a concave mirror. If you turn the spoon around, the outer surface acts as a convex mirror, a reflective surface with edges that curve away from the observer. What do you see when you look at the back of a spoon? You see an upright, but smaller image of yourself.
Properties of a spherical convex mirror are shown in Figure 5. Rays reflected from a convex mirror always diverge. Thus, convex mirrors form virtual images. Points F and C are behind the mirror. In the mirror equation, f and di are negative numbers because they are both behind the mirror.
The ray diagram in Figure 5 represents how an image is formed by a spherical convex mirror. The figure uses two rays, but remember that there are an infinite number of rays. Ray 1 approaches the mirror parallel to the principal axis. The reflected ray is drawn along a sight line from F through the point where ray 1 strikes the mirror. Ray 2 approaches the mirror on a path that, if extended behind the mirror, would pass through F.
The reflected part of ray 2 and its sight line are parallel to the principal axis. The two reflected rays diverge, and the sight lines intersect behind the mirror at the location of the image. An image produced by a single convex mirror is a virtual image that is upright and smaller compared to the object.
The magnification equation is useful for determining the apparent dimensions of an object as seen in a spherical convex mirror. If you know the diameter of an object, you can multiply by the magnification fraction to see how the diameter changes. You will find that the diameter is smaller,
as are all other dimensions. This is why the objects appear to be farther away than they actually are for convex mirrors.
Field of view
It may seem that convex mirrors would have little use because the images that they form are smaller than the objects. However, this property of convex mirrors does have practical uses. By forming smaller images, convex mirrors enlarge the area, or field of view, that an observer sees, as shown in Figure 6. Also, the center of this field of view is visible from any angle of an observer off the principal axis of the mirror;
thus, the field of view is visible from a wide perspective. For this reason, convex mirrors often are used in cars as passenger-side rearview mirrors.
Mirror Comparison
How do the various types of mirrors compare? Table 1 compares the properties of single-mirror systems with objects that are located on the principal axis of the mirror. Virtual images are always behind the mirror, which means that the image position is negative. When the absolute value of a magnification is between zero and one, the image is smaller than the object. A negative magnification means the image is inverted relative to the object. Notice that the single plane mirror and convex mirror produce only virtual images, whereas the concave mirror can produce real images or virtual images. Plane mirrors give simple reflections, and convex mirrors expand the field of view. A concave mirror acts as a magnifier when an object is within the focal length of the mirror.